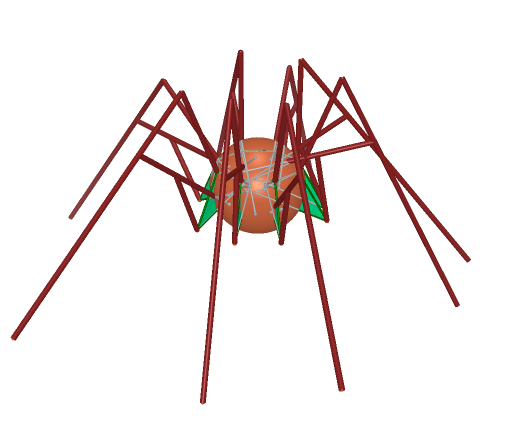
The Klann linkage is a planar mechanism designed to simulate the gait of legged animal and function as a wheel replacement. The linkage consists of the frame, a crank, two grounded rockers, and two couplers all connected by pivot joints.
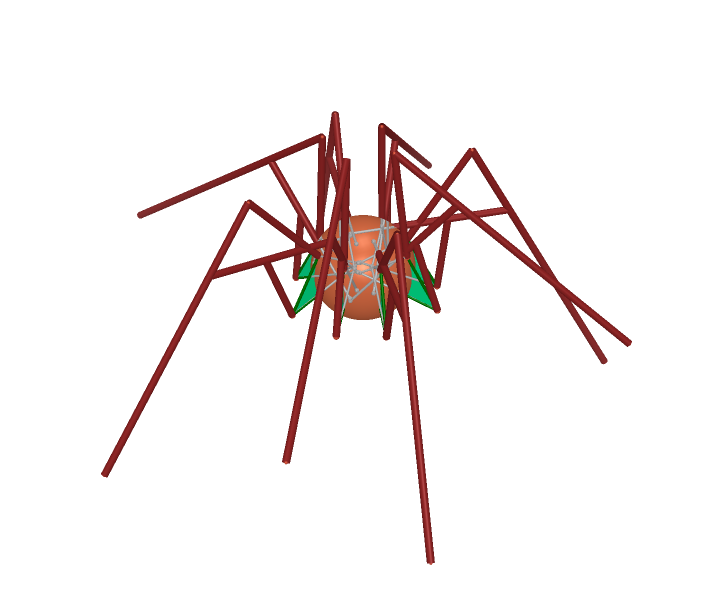
The picture above show the original model, and another has other four leg to make it more like a real spider. Click right pictures to see the animation. The following is a detailed construction process. There is not construction process of first picture since it is contained in the second one.
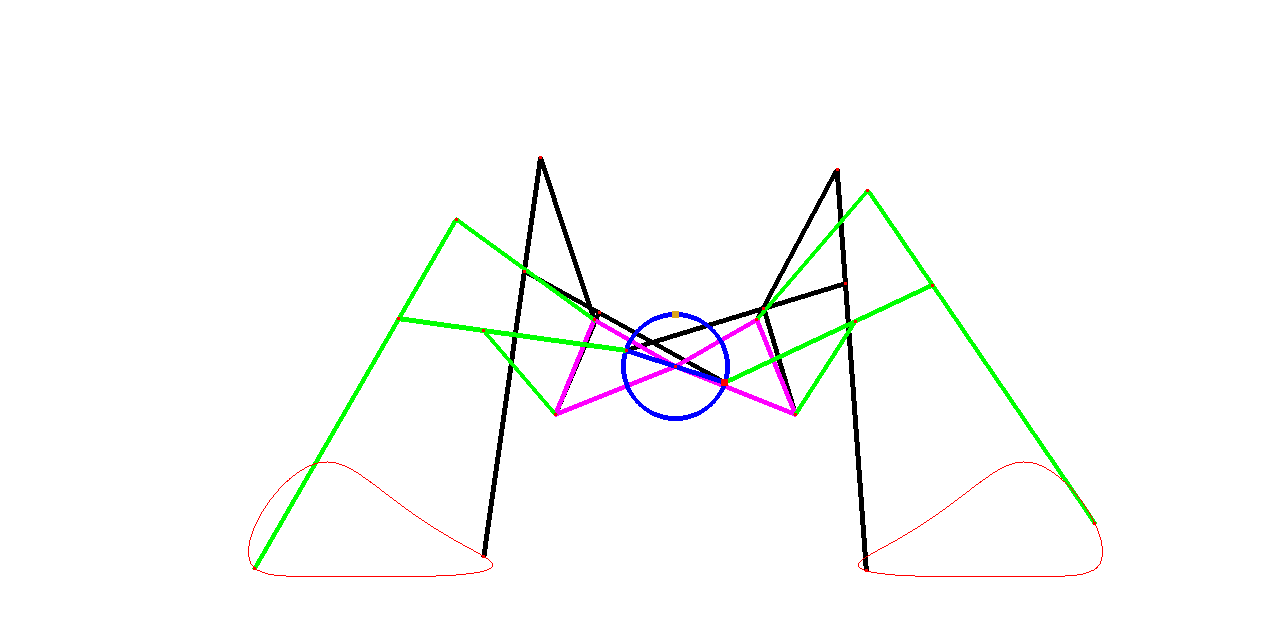
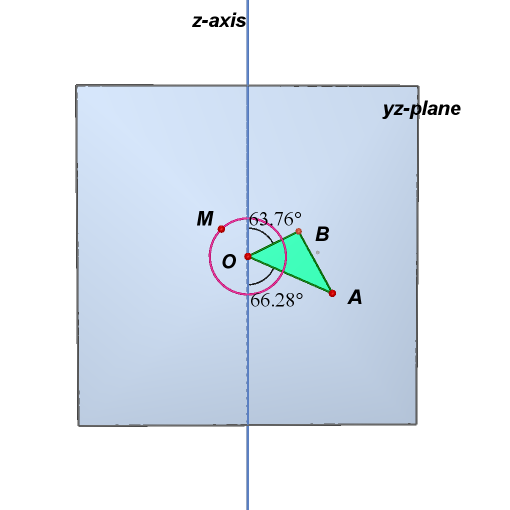
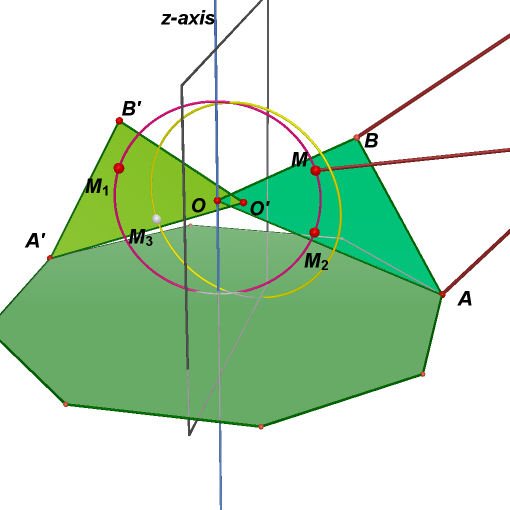
1. Start with a triangle
OAB in yz-plane where the angle between z-axis and segment OB is 63.76
degrees, the angle between z-axis and segment OA is 66.28 degrees, and
=
0.611 
.
2. Draw a circle in yz-plane centered on point O of radius 0.412
.
Take a movable point M on the circle.
3. In yz-plane, draw
circle centered on M of radius 1.143
and
circle centered on A of radius 0.909
.
Point C is the intersection of the two circles.
.
Point D is their intersection.
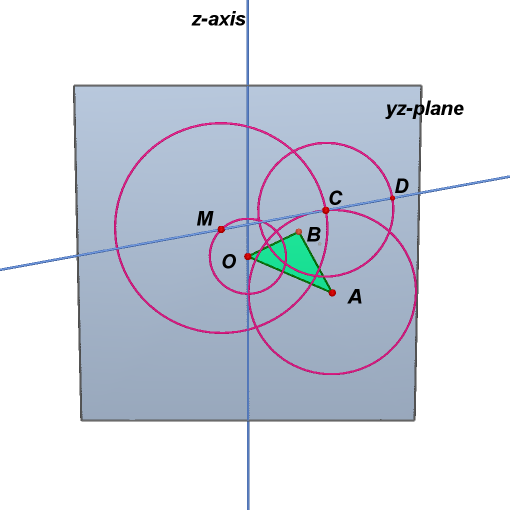
5. Hide previous three circles and line. Connect segment AC and MD.
6. In yz-plane, draw
circle centered on D of radius 0.93
and
circle centered on B of radius 1.323
.
Point E is the intersection of the two circles.
7. Create a line through E
and D and a circle centered on D in yz-plane of radius 2.577
.
Point F is their intersection.
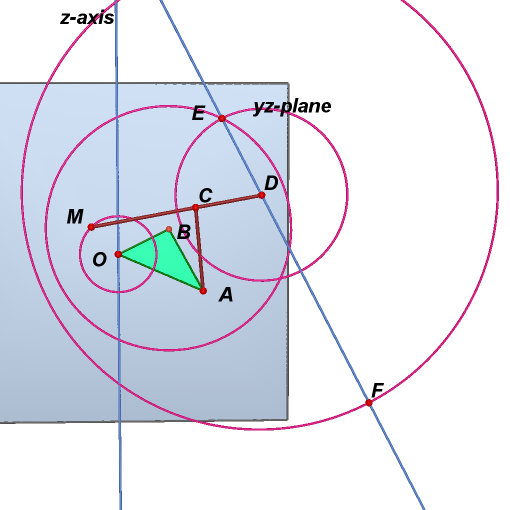
8. Hide previous three circles and line. Connect segment BE and EF.
We say that the four segments AC, MD, BE, EF, and triangle OBA compose the first leg.
9. Point M' is the central symmetry of point M through point O. Triangle OA'B' is the half-turn of triangle OAB around z-axis.
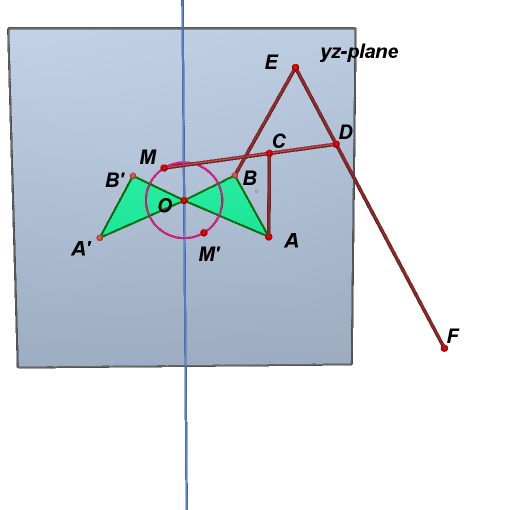
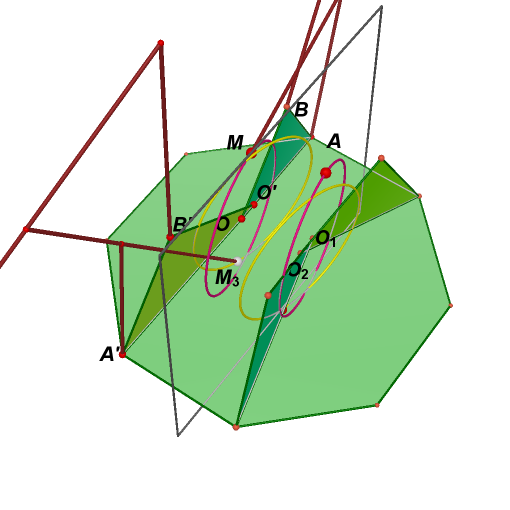
10. Hide yz-plane. Draw a regular octagon around z-axis through point A. Point G and H are adjacent vertices in the regular octagon, and point I is their midpoint.
11. Create a line parallel z-axis through point A. Rotate previous regular octagon around this line mapping point G to point I to obtain another regular octagon.
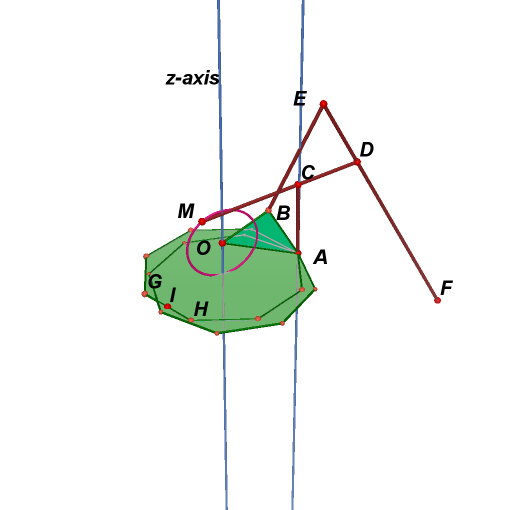
12. Hide the original regular octagon.
13. Create a plane bisecting the remaining regular octagon. Reflect triangle OAB and remaining circle in this plane to obtain a new triangle O'A'B' and a new circle.
14. Point
is
half turn of point M around z-axis. Point 
is
the central symmetry of
through
point O. Point
is
the reflection of point
in
bisecting plane.
15. Hide the bisecting
plane. Repeat steps 3~7 to point
and
triangle O'A'B'.
16. Create another
bisecting plane of regular octagon. Reflect two triangles and two
circles to obtain two new triangles and circles. Call the two vertices
of the triangle in the circle
,
.
17. Translate point M mapping point O to
.
Translate point
mapping
point O' to
.
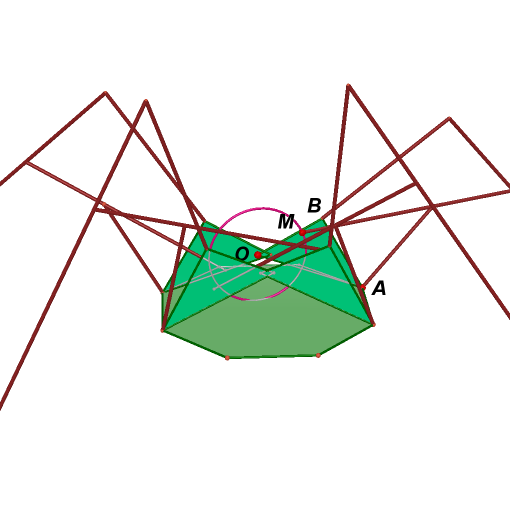
19. For convenience, we leave the first leg, and hide three other legs temporarily.
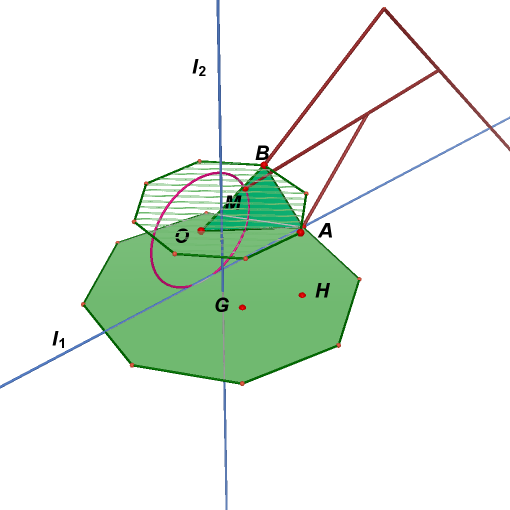
20. Create a line
which
is the intersection of the plane containing regular octagon and the
plane containing triangle OAB. Rotate point O and M 90 degrees
counterclockwise around this line to obtain point G and H.
21. Create a line
perpendicular
to regular octagon through its center. Draw a new regular octagon around
this line through point B.
22. Point I is a vertex of
new regular octagon obtained from rotating point B around line
clockwise
90 degrees. Create a line
through
point I and center of new regular octagon.
23. Take a point J on line
such
that
=
.
Translate point H mapping point G to point J to obtain point K.
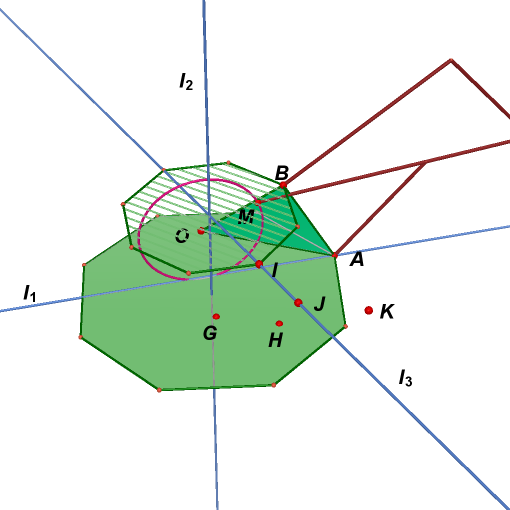
24. Hide point G, point H,
line
,
and bigger regular octagon.
25. Create two line in the
plane containing smaller regular octagon. One is perpendicular to line
through
point J, and another is parallel to line
through
point K. Call the intersecting point of two lines L.
26. Draw a line
parallel
line
through
point I. Create a plane P containing this line and midpoint between J
and L.
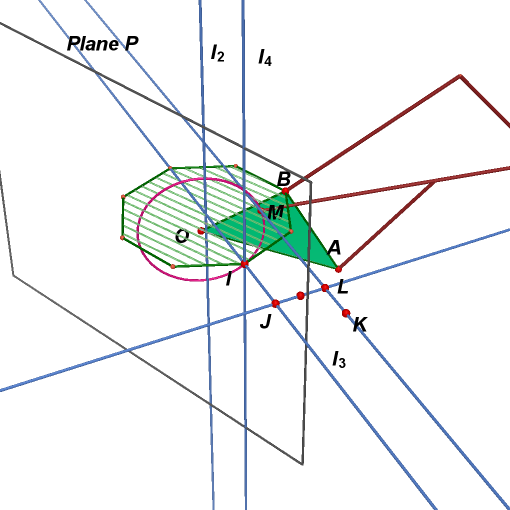
27. Hide point J, K, L,
line
,
and two line at step 22.
28. Translate point M and
triangle OBA to obtain point 
and
a new triangle. Point A'' is the vertex of new triangle corresponding to
point A.
29. Rotate point
and
new triangle around line
mapping
point A'' to an arbitrary point on plane P to obtain point
and
triangle T.
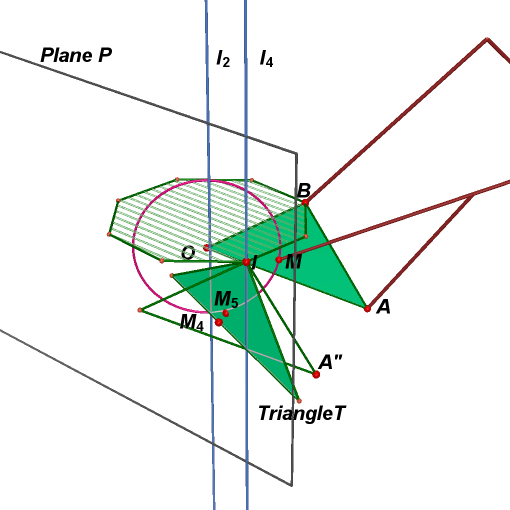
30.
Hide point
,
line
,
and triangle containing point A''. Repeat steps 3~7 to point
and
triangle T.
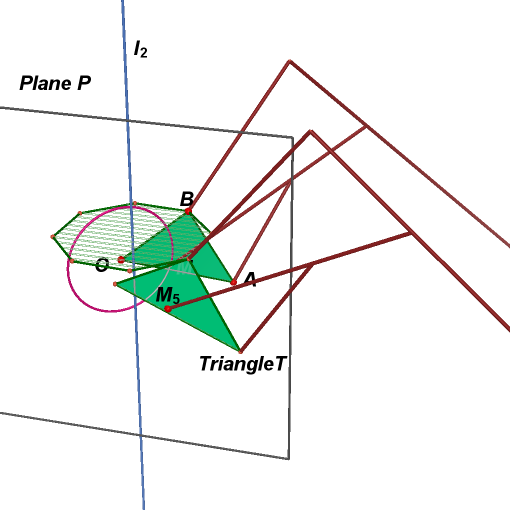
31. Create a plane
containing line
and
through point B. Reflect point
and
triangle T in this plane to obtain point
and
a new triangle T'. Also reflect plane P in this plane to obtain plane
P'.
32. Draw a line parallel
through
the vertex of triangle T' closest line
.
Take a point
half-turn
of point
around
this line.
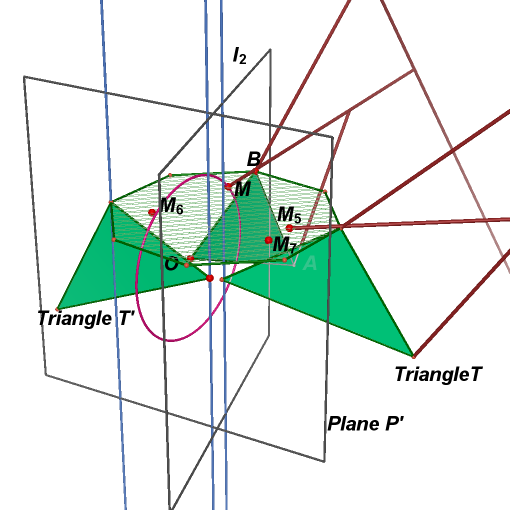
33. In plane P', repeat
steps 3~7 to point
and
triangle T'. Hide useless objects.
34. Rotate plane P',
triangle T' and point
around
line
135
degrees counterclockwise to obtain plane P'', triangle T'' and point
.
35. Take a point
central
symmetry of point
.
through the vertex of triangle T'' closest line
.
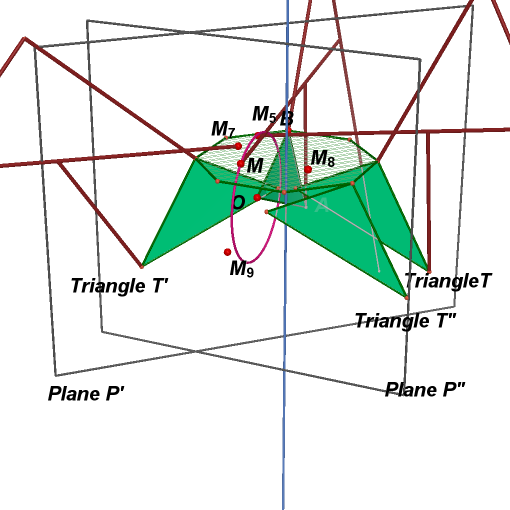
36. In plane P'', repeat
steps 3~7 to point
and
triangle T''. Hide the useless objects.
37. Create a plane perpendicular line
through
the midpoint between A and B. Point N is the intersection of
and
this plane. 
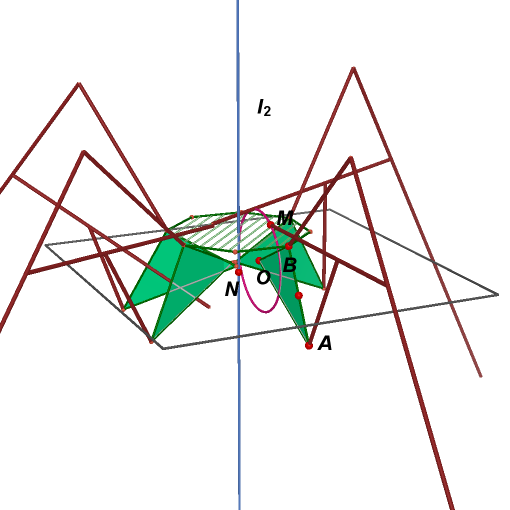
38. Repeat steps 31~32 to
point
and
triangle T to obtain final leg.
39. Show previous hidden three leg. Draw a sphere centered on point N through point B.
